Four-Color Theorem

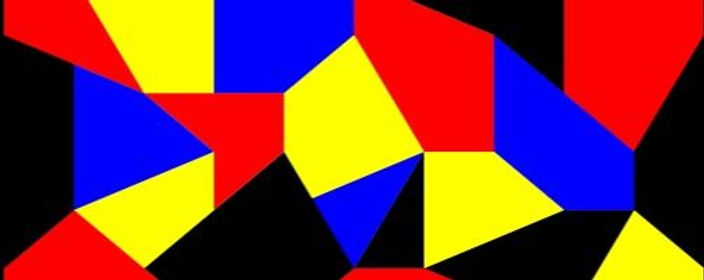
The four-color theorem was first discovered in 1852 by a man named Francis Guthrie, who at the time was trying to color a map of the UK. He found out that a maximum of four colors was all he needed such that no countries sharing a common boundary curve segment were of the same color. After making this conjecture, it was not until 1976 that he first published a correct proof by using minimality, and through which he eventually deduced the four-color theorem-- any map in a plane can be colored using four colors in such a way that no adjacent regions have the same color. Later, this theorem extended to other graph theories such as the five-color theorem and even to the infinite graphs, for which every finite subgraph is planar.